BQ#7: Unit V: Deriving the Difference Quotient

Deriving the difference quotient? ..What? ...Well, si se puede!

So, first of all let's recall the difference quotient formula and remember what it has in the entire thing. 

(http://www.coolmath.com/algebra/15-functions/images/08-functions-01.gif)

Remember the song for it? Yes! It is said like this: "f of x plus h, minus f of x, divided by the letter h that's the difference quotient!" (in rhythm) 

If we take a simple, regular (x,y) graph that we are familiar with, it can be somewhat easier to  understand..to begin with. Now, like the picture below, we have a point there and instead of being 1 or 5 like a scale we would normally see, we can just call it 'x'. Then, that means that the y value would have to be f(x). If you find it confusing then think of it with numbers to make sense out of it. If we had 1 instead of x, then the y value would have to be f(1) or even if we had 5 as the x value, then the y would be f((5). Making more sense? Hopefully, so now we can continue. Like shown below, if we had the next measurement it would be x+h and the reasoning is this: if we say that the distance from x, the first point, to the second point is of h value, then our second x point would be x+h. That means that the y-value for x+h would be f(x+h). 

Almost finishing up, we would have to use the slope formula in order to continue and the formula is: 

(http://0.tqn.com/d/create/1/0/9/p/C/-/slopeformula.jpg)

The derivative is basically slope and when we are looking for the derivative, then that just means we are looking for the slope of the horizontal tangent/secant line. The two coordinates that we found we can use to plug in to the slope formula. The visual is in the image below. Okay lastly, we should clear up what in the world is a tangent and a secant line? Well, a secant line is one of which touches the graph at two point while tangent lines touch the graph at one point only. 

Still need more help and I spoke gibberish, then go ahead and watch the video below:

            (https://www.youtube.com/watch?v=XA0fZh8cXV8)

References:

(http://www.coolmath.com/algebra/15-functions/images/08-functions-01.gif)

(http://0.tqn.com/d/create/1/0/9/p/C/-/slopeformula.jpg)

(https://www.youtube.com/watch?v=XA0fZh8cXV8)

BQ #6: Unit U

1. What is continuity? What is discontinuity?

Continuity basically means that the graph will be predictable, it will have NO breaks, NO jumps, and NO holes, it can be drawn without lifting your pencil, and lastly, the value (actual height) and the limit (intended height) will be the same. In other words what this means is that the graph will go where you think it will go and nothing will be there to interrupt the graph, so no holes, jumps, etc. For example, the picture below.

                                                     (http://www.conservapedia.com/images/2/2f/Br-cont-function.png)

Okay, so now what about a discontinuity? Well a discontinuity will have to be where a function is NOT continuous meaning that it will have breaks, jumps, holes, it will not be predictable, and the limit and the value of the graph will not be the same and equal to each other. We are aware that there are two types of families for the functions that would be removable and non-removable. There is only one removable function and that is the point (hole) discontinuity. The point discontinuity is the only on that has limit as well while the three that we non-removable do not have a limit, or said properly, the limit does not exist at the non-removable discontinuities. These non-removable are jump discontinuity, oscillating behavior, and infinite behavior. Now, the reason that the limit does not exist is due to the fact that in a jump there is a different left and right and the do not meet, in oscillating behavior,...well that thing is just going up and down and it is just confused, and lastly, the infinite behavior means that there is a vertical asymptote which leads to unbounded behavior and also, infinity is not a real number to be dealing with.

This image is an example of a point (hole) discontinuity:

                                        (http://faculty.wlc.edu/buelow/common/imageN20.JPG)

This image is an example of a jump discontinuity:

(http://image.tutorvista.com/content/feed/u364/discontin.GIF)

This image is an example of oscillating behavior:

(http://webpages.charter.net/mwhitneyshhs/calculus/limits/limit-graph8.jpg)

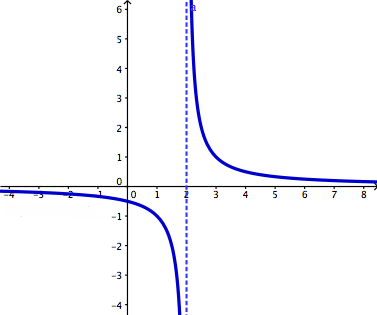
This image is an example of infinite discontinuity:

(http://dj1hlxw0wr920.cloudfront.net/userfiles/wyzfiles/44bad38c-431e-4382-8fe9-86303561b2a0.gif)

2. What is a limit? When does a limit exist? When does a limit not exist? What is the difference between a limit and a value?

A limit is the INTENDED height of a function. A limit exists only when the left side and the right side of the graph meet. Sometimes the circle on the graph is not shaded in and it an open circle, so take a point discontinuity..that's a perfect example because a limit still exist at the point since a limit is only the INTENDED value and that is where the graph is intending to go. Now, at the other three discontinuities the limits do not exist and that is because for some reason the left and right do not match and meet, there is oscillating behavior, and/or there is infinite behavior. The main difference between a limit and a value is that the limit is the INTENDED height of a function, while a value is the ACTUAL height of a function.

3. How do we evaluate limits numerically, graphically, and algebraically?

To evaluate the limit numerically, you would take a t-table of x and y values. We begin by putting the number they give us that it is approaching in the middle of the table and then fill the left and right with the closest numbers that head to the one in the middle. That is actually the most confusing and most difficult because then you can just take your calculator and plug in the numbers to be able to hit trace and basically then just copy down the numbers. For example:


(http://archives.math.utk.edu/ICTCM/VOL10/C006/image2.gif)

To evaluate the limit graphically, we simply put a finger on the left and a finger on the right and follow the graph until your fingers meet ...well...if they meet. Remember they will not always meet because it can be a jump, etc. For example take the graph below and see how if you trace the graph your fingers will not meet because it is a jump and they are different values...this is not like a point of which your fingers would meet.

(http://www.vitutor.com/calculus/limits/images/0_268.gif)

Lastly, to evaluate a limit algebraically, is well you are using equations and we solve. The methods we can use are direct substitution, dividing out/factoring method, and the rationalizing/conjugate method.

Works Cited:
http://www.conservapedia.com/images/2/2f/Br-cont-function.png

http://faculty.wlc.edu/buelow/common/imageN20.JPG

http://image.tutorvista.com/content/feed/u364/discontin.GIF

http://webpages.charter.net/mwhitneyshhs/calculus/limits/limit-graph8.jpg

http://dj1hlxw0wr920.cloudfront.net/userfiles/wyzfiles/44bad38c-431e-4382-8fe9-86303561b2a0.gif

http://archives.math.utk.edu/ICTCM/VOL10/C006/image2.gi

http://www.vitutor.com/calculus/limits/images/0_268.gif

BQ#3: Unit T Concepts 1-3: Trig Graphs

How do the graphs of sine and cosine relate to each of the others? Emphasize asymptotes in your response. 
-Tangent
-Cotangent
-Secant
-Cosecant 

1) Tangent 
     Okay so the ratio identity for tangent we know is sine/cosine. Also, we are aware that we will only have asymptotes where we get an undefined answer meaning that we divide by zero and therefore, cosine would have to be zero in order to make this asymptotes and an undefined answer. Now, we will have asymptotes at the 90 and 270 degree, so at pi/2 and 3pi/2. This being said, we know that tangent cannot touch them no matter what. Now, there are way more asymptotes, they practically go on forever, yet we can just stick to some.

2) Cotangent 
        We can basically use the same knowledge from above with Tangent, yet now we know that cotangent is equaled to cosine over sine and so the asymptotes will be found where cosine is zero so at 90 and 270 degrees, or pi/2 and 3pi/2. And we remember that the graph cannot touch the asymptotes!

3) Secant 

      We know that secant is the reciprocal of cosine and the ratio is 1/cos. Secant as well has asymptotes that are found where cosine is equaled to zero as well. 

4) Cosecant 

      Now, the same rules apply like with the reciprocals and stuff yet the asymptotes will be located in different places due to the different kind of graph. Cosecant is the reciprocal of sine so its ratio is 1/sin. 

References:

http://mathsm1m0838.edublogs.org/files/2009/08/Picture-11.png

http://2.bp.blogspot.com/-KGMdRWBNlP8/UXi7SKh2BjI/AAAAAAAAAEU/YSex9MpWZAk/s640/2013-04-24+22.05.53-1.jpg

http://www.mathipedia.com/GraphingSecant,Cosecant,andCotangent_files/image014.jpg

http://www.ask-math.com/images/Cosecantgraph.png

BQ#4: Unit T Concept 3: Tangent and Cotangent Graphs

Why is the "normal" tangent graph uphill, but a "normal" cotangent graph downhill? Use unit circle ratios to explain.
        
        The reason that a tangent graph is uphill and yet a cotangent graph is downhill is due to the fact that they are different ratios and different of which then creates different asymptotes. We know that tan=sin/cos and cot=cos/sin, or in other words, tan is equaled to y/x and cot is equaled to x/y. See how the denominators are different, tangent has a denominator of cosine while cotangent has a denominator of sine and therefore for tangent we would get our asymptotes wherever cosine is equaled to zero which would be at the 90 degree (pi/2) and at the 270 degree (3pi/2). Furthermore, for cotangent we would get asymptotes wherever sine is equaled to zero which would be at the 0 degree (0) and at the 180 degree (pi). We do know that the pattern for both is positive, negative, positive, negative, yet the asymptotes are located in distinct places and so creates a different looking graph.   

References:

-http://www.mathamazement.com/images/Pre-Calculus/04_Trigonometric-Functions/04_06_Graphs-of-Other-Trig-Functions/cotangent-graph.JPG

-http://www.regentsprep.org/Regents/math/algtrig/ATT7/otherg91.gif

BQ#5: Unit T Concepts 1-3

Why do sine and cosine NOT have asymptotes, but the other four trig graphs do? Use unit circle ratios to explain. 
     The reason that sine and cosine cannot have asymptotes is due to the fact that they will never be undefined. Now, let's recall that in order for there to any asymptotes then we have to have an answer as undefined. Okay, with that in mind we can now prove that sine and cosine do NOT have any asymptotes. We know that the ratio for sine is y over r and as for cosine, we know that it is x over r. "R" is always equaled to one right? And since we will never have a zero be on the denominator, then that means that no asymptotes will be present and created. 
      
     The other four trig graphs do have asymptotes and that is because they will not ever have r or a 1 as the denominator. For example, if we did tangent, then we know the ratio is sine (y) over cosine (x) and we know that x can be a zero at the 90 degrees and at the 270 degrees, or pi and 3pi over 2. Also for secant, it would be the same thing since the ratio is r over x and x can be a zero like stated before. Lastly, cosecant (r/y) and cotangent (x/y), they have a denominator of y and we know that y can be equaled to zero at 0 degrees and 180 degrees, or in other words, 0 and pi.  

BQ#2: Unit T Concept Intro: Trig Graphs and the Unit Circle

How do trig graphs relate to the Unit Circle?
   Okay so I know what you are thinking..the Unit Circle again?! Well yes, the Unit Circle comes in from everywhere and somehow has a function for everything. For trig graphs, it is as if you are seeing the Unit Circle, yet in a straight line and the same numbers are present in order. Also, their signs depend on what quadrant they are found in. For example, if we have cosine, we know that it is positive in quadrants 1 and 4.

1. Period? Why is the period for sine and cosine 2pi, whereas the period for the period for tangent and cotangent is pi?
    The period for sine and cosine is 2pi due to the pattern that it follows and how it repeats after every four marks on a graph. Now, for tangent and cotangent and we are able to see that it repeats every two marks. For proof we can see it after knowing that the symbols are positive, negative, positive, negative and so that means that it only takes two marks. 

2. Amplitude?-How does the fact that sine and cosine have amplitudes of one (and the other trig functions don't have amplitudes) relate to what we know about the Unit Circle?
    So first of all, what are amplitudes? Well an amplitude is half the distance between the highest and lowest points on the graph. They can be found by looking at the value of |a|. Sine and cosine have the same amplitude of which is 1 and -1 due to the fact that they are the ones with the restrictions. They cannot be any points greater than or less than 1 and -1 since they don't exist anymore. Now, we get to tangent and cotangent. They have no restrictions and therefore can keep going on and on and on. Their range will always be negative infinity to positive infinity. Lastly, we have cosecant and secant of which simply don't have a range and therefore no amplitudes. 

Reflection#1: Unit Q: Verifying Trig Functions

Dear Future Math Students,

Unit Q deals with several fundamental identities, trig functions, ratios, and using different simplification processes in order to solve and verify as well. I know that this sounds like gibberish and that you probably want to cry because it just seems so confusing and you just do not want to go through this, but DON'T WORRY! I did it and it was okay....after you practice practice practice and get the feel for it. I am not going to lie, IT IS DIFFICULT! But it will not be the end of the world! Do not give up and try and practice and sooner or later you will succeed! (: Okay well let me go more in depth...


1. What does it actually mean to verify a trig identity?
-To verify simply means to check and prove that whatever is stated is true. For example, if you had 1+1=2 then you simply have to go through steps that will help you prove that 1+1 actually does equal 2. Of course, it will not be a nice and easy as my example. ...Sorry ._. And so, basically that you have to be sure to solve a problem and get the same answer as the one that is given and equaled to. Oh! and it is better when you have to verify rather than having to just solve and come up with an answer. The reason is that when you are verifying, you know the answer you should end up with and it is just you having to plug in and substitute and solve until you hit bingo and check the answer, whereas when it comes to just solving and you are obviously not given an answer, then you just have to solve correctly and pray you get it correct.

2. What tips and tricks have I found helpful?
-Okay so we know that there are a bunch of functions and ratios like sine, cosine, tangent, cosecant, secant, and cotangent, yet a tip I have is to turn everything into sin/cos at some point because it will become easier. Sine and cosine can be considered our best friends in this case. They are nice to us, so might as well use them. (; Also, I have foreseen the future and I know that you will feel discouraged and defeated, but NO! You can do this! DON'T GIVE UP! This is a tip that I know you WILL need! So use it! Keep working through it and take various adventures with the problem.

3. Explaining my thought process and steps I take in verifying a trig identity.
-Okay so if you noticed, I said that you should take various adventures with the problem and what did I mean? Well the beauty of these problems is that there isn't only one way on solving a problem so you really can't o wrong! Yay! If you begin differently than someone else, it's okay just keep going and don't get stuck, use your knowledge, you identities, your reciprocals. Now, when I encounter a problem I think and wonder what I should do first. Should I turn into sine and cosine? Should I multiply by the conjugate? Should I split the problem up maybe to see if something cancels? Or maybe I should find the least common denominator? Perhaps this is an identity? Honestly, I just think of what to do first and then I keep going just trying stuff and see where it takes me and I just use the skills I know. There is never one path so don't be afraid of trying something! (: