Create your own Playlist on MentorMob!
Thursday, December 19, 2013
Tuesday, December 17, 2013
WPP#9: Unit L Concepts 5-8
Create your own Playlist on MentorMob!
Sunday, December 8, 2013
SP #6: Unit K Concept 10
Although these types of problems seem the easiest, they can become the trickiest of all due to the fact that there are several phases of which you can make silly mistakes. It is important to pay attention when it comes to knowing how many zeros go after the decimal and also when it comes to the dividing since they are fractions. You have to remember to multiply by the reciprocal and then another important part is making sure whether there is a number in front of the decimal like in this case. Remember to add it in the end and do not just forget like I almost did.
Sunday, December 1, 2013
Fibonacci Beauty Ratio (Extra Credit)
Name: Leslie N.
Foot to navel: 97 cm Navel to top of head: 63 cm Average: 1.577
Navel to chin: 45 cm Chin to top of head: 21 cm
Knee to navel: 48.5 cm Foot to knee: 46 cm
Name: Rodolfo R.
Foot to navel: 103 cm Navel to top of head: 68 cm Average: 1.547
Navel to chin: 46.5 cm Chin to top of head: 22 cm
Knee to navel: 54 cm Foot to knee: 53 cm
Name: Michael C.
Foot to navel: 110 cm Navel to top of head: 66 cm Average: 1.84
Navel to chin: 46.5 cm Chin to top of head: 18 cm
Knee to navel: 65 cm Foot to knee: 51 cm
Name: Omar T.
Foot to navel: 113.5 cm Navel to top of head: 67 cm Average: 1.687
Navel to chin: 50 cm Chin to top of head: 22 cm
Knee to navel: 62 cm Foot to knee: 56.5 cm
Name: Leslie E.
Foot no navel: 107 cm Navel to top of head: 58.5 cm Average: 1.81
Navel to chin: 43 cm Chin to top of head: 17 cm
Knee to navel: 53 cm Foot to knee: 49 cm
Throughout this activity, the goal was to see who was the most mathematically beautiful by taking some measurements and then finding the average. In the end, who ever was the closet to 1.618 or anyone who actually hit that decimal was the most beautiful. As I measured and then found the average I realized that the closest one was Leslie N. I think so she was the closest to being the "most beautiful".
I believe that the Fibonacci Beauty Ratio is not anything that should be considered when it comes to beauty. It seems rather stupid to me and just is not reasonable, everyone is beautiful in their own ways and this can just bring some people down. I do not think that people would use this method in order to see if a person was beautiful or not.
Foot to navel: 97 cm Navel to top of head: 63 cm Average: 1.577
Navel to chin: 45 cm Chin to top of head: 21 cm
Knee to navel: 48.5 cm Foot to knee: 46 cm
Name: Rodolfo R.
Foot to navel: 103 cm Navel to top of head: 68 cm Average: 1.547
Navel to chin: 46.5 cm Chin to top of head: 22 cm
Knee to navel: 54 cm Foot to knee: 53 cm
Name: Michael C.
Foot to navel: 110 cm Navel to top of head: 66 cm Average: 1.84
Navel to chin: 46.5 cm Chin to top of head: 18 cm
Knee to navel: 65 cm Foot to knee: 51 cm
Name: Omar T.
Foot to navel: 113.5 cm Navel to top of head: 67 cm Average: 1.687
Navel to chin: 50 cm Chin to top of head: 22 cm
Knee to navel: 62 cm Foot to knee: 56.5 cm
Name: Leslie E.
Foot no navel: 107 cm Navel to top of head: 58.5 cm Average: 1.81
Navel to chin: 43 cm Chin to top of head: 17 cm
Knee to navel: 53 cm Foot to knee: 49 cm
Throughout this activity, the goal was to see who was the most mathematically beautiful by taking some measurements and then finding the average. In the end, who ever was the closet to 1.618 or anyone who actually hit that decimal was the most beautiful. As I measured and then found the average I realized that the closest one was Leslie N. I think so she was the closest to being the "most beautiful".
I believe that the Fibonacci Beauty Ratio is not anything that should be considered when it comes to beauty. It seems rather stupid to me and just is not reasonable, everyone is beautiful in their own ways and this can just bring some people down. I do not think that people would use this method in order to see if a person was beautiful or not.
Monday, November 25, 2013
"Fibonacci Haiku: Si Para Tu"
Sunday, November 17, 2013
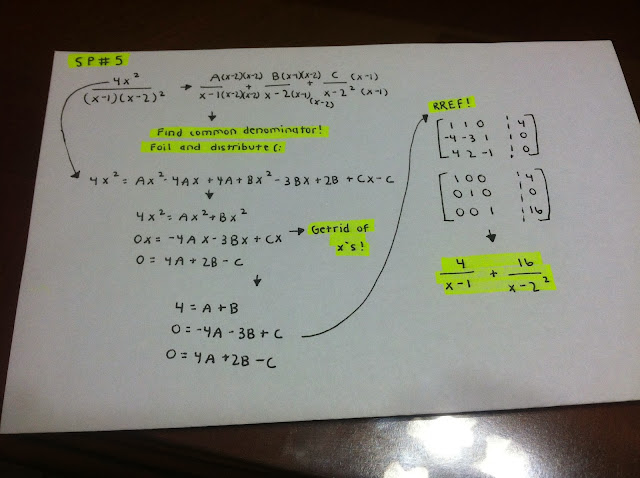
SP #5 Unit J Concept 6
SP #5 (:
While solving this problem, it is similar to the other and you have to be cautious when it comes to the foiling and the distributing as well. Also, do not forget to get rid of the x's when towards the end because if not you will not be able to continue solving. Be sure to solve properly and then use RREF to solve fully. Plug in the numbers correctly and make sure to know how to use the different buttons and the next steps.
While solving this problem, it is similar to the other and you have to be cautious when it comes to the foiling and the distributing as well. Also, do not forget to get rid of the x's when towards the end because if not you will not be able to continue solving. Be sure to solve properly and then use RREF to solve fully. Plug in the numbers correctly and make sure to know how to use the different buttons and the next steps.
SP #4: Unit J Concept 5
SP #4 :)
When solving this equation it is important to be careful when foiling and distributing properly while searching for the common denominator. Also, since there are several steps to the problem you should just be aware of what you are doing and the steps that come next. When combining like terms it can get confusing so it is best to do it all out in paper and carefully. Lastly, know how to use the calculator and be sure to plug in the numbers correctly.
Monday, November 11, 2013
SV #5 Unit J Concepts 3-4
What does the viewer need to pay special attention to in order to understand?
The viewer has to be very cautious when it comes to making sure they place the numbers correctly and also when it comes to multiplying, adding, and subtracting correctly. The reason is because that is when many times silly mistakes are made. Another thing is that you should write down every step made because if you make a mistake it will be easier to find it when looking back at what it is you had done so far. Make sure to highlight or just be aware of what it is you are trying to achieve, for example make sure you are looking for the correct zero and placing it where it belongs. You basically have to be aware of every move you do andmake sure you are doing it properly.
The viewer has to be very cautious when it comes to making sure they place the numbers correctly and also when it comes to multiplying, adding, and subtracting correctly. The reason is because that is when many times silly mistakes are made. Another thing is that you should write down every step made because if you make a mistake it will be easier to find it when looking back at what it is you had done so far. Make sure to highlight or just be aware of what it is you are trying to achieve, for example make sure you are looking for the correct zero and placing it where it belongs. You basically have to be aware of every move you do andmake sure you are doing it properly.
Monday, October 28, 2013
Sunday, October 27, 2013
SV #4 : Unit I Concept 2- Graphing logarithmic functions and identifying factors
SV #4
What does the viewer need to pay special attention to in order to understand?
The viewer should pay attention to the fact that in this concept you use x=h instead of y=k. This one is slightly different than concept 1 and so the viewer should be cautious about that. Also, for the domain and range, it is as if they switch places compared to the last concept. The reason is because in this case the range is always all real numbers and then for the domain, it will basically always be the asymptote and then comma to infinity since we are always going to be focused to the right side of the asymptote. Lastly, when it comes to solving for the x and y intercepts just be aware and know how to solve properly.
What does the viewer need to pay special attention to in order to understand?
The viewer should pay attention to the fact that in this concept you use x=h instead of y=k. This one is slightly different than concept 1 and so the viewer should be cautious about that. Also, for the domain and range, it is as if they switch places compared to the last concept. The reason is because in this case the range is always all real numbers and then for the domain, it will basically always be the asymptote and then comma to infinity since we are always going to be focused to the right side of the asymptote. Lastly, when it comes to solving for the x and y intercepts just be aware and know how to solve properly.
Thursday, October 24, 2013
SP # 3: Unit I Concept 1
The equation is:
F(x)= -2(2)^(x+2)-1
For this problem, the first step that we would do is identify a-k and then the asymptote becomes easy (y=k). Then, finding the x and y intercepts would come and we would do so by plugging y as zero when solving for the x intercept and then plugging in zero for x to find the y intercept. The domain and range you can either do before or after graphing the equation. Plug in any key points, just make sure that the third one is your value of h to make it easy. Plot the points and then you are basically done.

What does the viewer need to pay special attention to in order to understand?
While solving the problem the viewer should pay special attention to the original equation and whether a indicates it as below or above the asymptote. This will come in handy for the graphing as well. Now, if and when you get a negative natural log then you have to realize and remember that it means it will be undefined. Remember that the domain is always all real numbers and then since in this case for the range it is below that means that you will start from negative infinity up to the asymptote.
F(x)= -2(2)^(x+2)-1
For this problem, the first step that we would do is identify a-k and then the asymptote becomes easy (y=k). Then, finding the x and y intercepts would come and we would do so by plugging y as zero when solving for the x intercept and then plugging in zero for x to find the y intercept. The domain and range you can either do before or after graphing the equation. Plug in any key points, just make sure that the third one is your value of h to make it easy. Plot the points and then you are basically done.

What does the viewer need to pay special attention to in order to understand?
While solving the problem the viewer should pay special attention to the original equation and whether a indicates it as below or above the asymptote. This will come in handy for the graphing as well. Now, if and when you get a negative natural log then you have to realize and remember that it means it will be undefined. Remember that the domain is always all real numbers and then since in this case for the range it is below that means that you will start from negative infinity up to the asymptote.
Wednesday, October 16, 2013
SV #3: Unit H Concept 7
SV #3
What does the viewer need to pay special attention to in order to understand?
While watching the video, the viewer should pay special attention when it comes to having the clues in mind due to the fact that when breaking down the fraction given you should know the way to simplify it. Also when it comes to expanding because several times you can forget that you maybe there should be a coefficient since a number appeared more than once. The mistakes that you can make can be rather silly ones so paying attention while working the problem out would be helpful.
Monday, October 7, 2013
SV #2 Unit G Concept 1-7: Finding Vertical/Horizontal Asymptotes, Slants, etc
What is this problem about?
This problem is about finding the vertical asymptotes as well as the horizontal asymptotes and just finding several parts to it. Slants are included as well and a fact you know is that if you have a horizontal asymptote then you do not have a slant or vice versa since you cannot have both in one equation. Graphing it is another step to this and you have to find the domain, x intercepts, and y intercepts as well. There are several steps in this Unit and it requires a lot of memorization. A tip would be studying the flashcards or rehearsing the chants so that you know what to do when looking for a slant or vertical asymptote.
What does the viewer need to pay special attention to in order to understand?
These types of problems are very tricky and can be easy when it comes to messing up and forgetting how to solve for a certain part. The reason is because there are several parts to it and several techniques on how to do it. Now, the viewer has to pay special attention to the rules because if they should realize the fact that if they find a horizontal asymptote, then they should not have a slant. Also, when it comes remembering what to do specifically to find a certain part. They should know exactly what to do when solving and for example know that if looking for a slant then long division is what to use.
This problem is about finding the vertical asymptotes as well as the horizontal asymptotes and just finding several parts to it. Slants are included as well and a fact you know is that if you have a horizontal asymptote then you do not have a slant or vice versa since you cannot have both in one equation. Graphing it is another step to this and you have to find the domain, x intercepts, and y intercepts as well. There are several steps in this Unit and it requires a lot of memorization. A tip would be studying the flashcards or rehearsing the chants so that you know what to do when looking for a slant or vertical asymptote.
What does the viewer need to pay special attention to in order to understand?
These types of problems are very tricky and can be easy when it comes to messing up and forgetting how to solve for a certain part. The reason is because there are several parts to it and several techniques on how to do it. Now, the viewer has to pay special attention to the rules because if they should realize the fact that if they find a horizontal asymptote, then they should not have a slant. Also, when it comes remembering what to do specifically to find a certain part. They should know exactly what to do when solving and for example know that if looking for a slant then long division is what to use.
Sunday, September 29, 2013
SV #1: Unit F Concept 10
What is this problem about?
This problem is about finding all of the zeroes including real and complex zeros as well when given a a polynomial of fourth or fifth degree. Basically, we will be looking for several components like the p's and q's. Also, we will solve for the positive and negative zeroes. In the end we will use synthetic division to find the zeroes with perhaps multiplicities and then once we break it down to a quadratic we can factor out usually with the quadratic equation.
What does the viewer need to pay special attention to in order to understand?
The view should pay special attention when it comes to the factorization. The reason is considering that it involves a lot of simplifying, it will not cut it if they are not fully paying attention. For example forgetting the number you take out when simplifying the equation and putting it into the factorization part to it is a common mistake. Another component is that many times you may forget to to do the extra step when simplifying complex numbers. These problems require full time of full attention.
Monday, September 16, 2013
SP #2 Unit E Concept 7: Graphing Polynomials
SP
What is this problem about?
This problem is basically about graphing a polynomial and being able to find several factors to it. For example, from the equation we have to factor it out and then find the zeros, or x-intercepts. Some times you can start off backwards, like in this case, by coming up with the zeros first and then the factored equation to finally foil. Finding the y-intercepts as well would a task and then the extrema with the intervals of increase and decrease. To be able and graph it, you look at the zeros and decide whether the graph will go through the point, bounce, or curve. The way you decide is by knowing what the multiplicities are.
The viewer needs to pay special attention to the x-intercepts and know what the multiplicities exactly due to the fact that the graph will come out incorrect if the multiplicities are wrong. Also, another thing is that knowing the end behavior is important since it will give you a hint of how the graph will look like. Just overall be cautious because one simple mistake can ruin the whole problem.
Wednesday, September 11, 2013
WPP #4 Unit E Concept 3: Finding maximum and minimum values of quadratic applications
Create your own Playlist on MentorMob!
Monday, September 9, 2013
WPP #3 Unit E Concept 2:Parts to Quadratics
Create your own Playlist on MentorMob!
SP #1 Unit E Concept 1: Identifying Parts of the Graph of a Quadratic
SP

1. What is this problem about?
This problem is about parent graphs and being able to graph them as well as find many different factors of the quadratic. For example, we are going to be finding the y-intercepts and x-intercepts algebraically. Also, finding the vertex is another point to this problem. We will be able and get to all of those answers by completing the square of the function.
2. What does the viewer need to pay special attention to in order to understand?
The viewer needs to pay special attention to the way that the parent function equation is written. The reason is because they have to make sure and it is not written in standard form. Another thing is that they have to be extra careful and be cautious when it comes to imaginary numbers.

1. What is this problem about?
This problem is about parent graphs and being able to graph them as well as find many different factors of the quadratic. For example, we are going to be finding the y-intercepts and x-intercepts algebraically. Also, finding the vertex is another point to this problem. We will be able and get to all of those answers by completing the square of the function.
2. What does the viewer need to pay special attention to in order to understand?
The viewer needs to pay special attention to the way that the parent function equation is written. The reason is because they have to make sure and it is not written in standard form. Another thing is that they have to be extra careful and be cautious when it comes to imaginary numbers.
Thursday, September 5, 2013
WPP #2 Unit A Concept 7: Revenue, Cost, and Profit
Create your own Playlist on MentorMob!
WPP #1 Unit A Concept 6: Linear Model
Create your own Playlist on MentorMob!
Subscribe to:
Comments (Atom)